Go to:

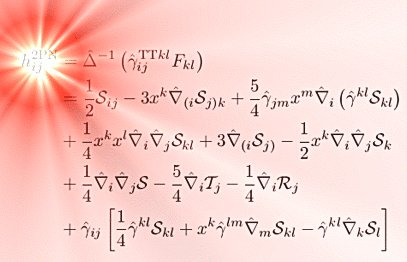-Introduction
-Simulations
-References

Relativistic Hydrodynamics
Gravitational Waveform Catalog

Departamento de Astronomía y Astrofísica

Universidad de Valencia

Theoretisch-Physikalisches Institut

Friedrich-Schiller Universität Jena

Departamento de Astronomía y Astrofísica

Universidad de Valencia

Departamento de Astronomía y Astrofísica

Universidad de Valencia

Theoretisch-Physikalisches Institut

Friedrich-Schiller Universität Jena

Numerical simulations of astrophysical scenarios are, like many studies based on computation, limited by several factors. Among these are not only our possible incomplete knowlegde of the governing laws of nature applicable to a specific scenario and its physical complexity, but also the computational speed and efficiency of the numerical simulation (in terms of wall-clock time, parallelizability, memory requirement, etc.) as well as numerical stability. The latter issue can pose severe problems to simulations of evolving systems, if the desired evolution time exceeds the time window of stability in the numerical simulation, i.e., if the numerical results become strongly inaccurate or even ill-defined before the final evolution time is reached.

This stability issue can have particularly unpleasant consequences in the case of numerical relativity. In many standard formulations, the Einstein field equations for the spacetime metric, which describes the gravitational field of, e.g., a star or a black hole, seem to have properties which makes a numerical evolution of them very unstable. A typical consequence of this is that after some evolution time the results of some constraint equations, which should remain zero at all times, start to grow. If the growth of this constraint violation is rapid (e.g., exponential) and the results thus strongly deviate from zero, the simulation can become very inaccurate and the computer code possibly crashes.

After decades of experimenting, scientists have discovered reformulations of the Einstein field equations, which are analytically equivalent to the numerically problematic ones, but exhibit much better stability properties. However, in many cases these alternative formulations need very complicated and/or time-consuming numerical algorithms to be solved on a computer. One possible way out of this dilemma is to neglect certain terms if they are small and thus unimportant in a specific astrophysical situation. Such an approximation of the full Einstein equations can then significantly reduce the complexity of the metric equations, while inheriting the advantageous stability properties of the original system.

One such approach is the one we have utilized in our previous numerical simulations of general relativistic rotational core collapse [Dimmelmeier, et al., 2001, Dimmelmeier, et al., 2002a, Dimmelmeier, et al., 2002b] in which the exact metric in the ADM spacetime foliation is approximated by assuming conformal flatness for the three-metric gamma_{i j}, which is also known as conformal flatness condition (CFC) or Isenberg-Wilson-Mathews approximation [Wilson, et al., 1996]:

gamma_{i j}^CFC = phi ⁴ gamma_{i j}^flat,

where phi is the conformal factor and gamma_{i j}^flat is the flat three-metric. Apart from simplifying the hydrodynamic and metric equations, this approach also exhibits numerical stability even for long evolution times, as it solves all constraint equations and thus cannot violate them by definition.

The CFC approximation can successfully be applied to simulations of rotational supernova core collapse, as in this context any deviations from conformal flatness in the spacetime metric are small. To be able to quantify these deviations, and to acquire a better approximation to the exact three-metric of the Einstein equations, we have introduced CFC+, an extension of the CFC appromimation which includes the transverse traceless deviation h_{i j}^2PN from CFC up to the second post-Newtonian order [Cerda, et al., 2005]:

gamma_{i j}^CFC+ = phi ⁴ gamma_{i j}^flat + h_{i j}^2PN.

Interestingly, in CFC+ the mathematical structure of CFC (fully constrained metric equations of elliptic character) is preserved. Therefore, the resulting evolution system of general relativistic hydrodynamics remains numerically stable. The equations for the additional metric components h_{i j}^2PN are linear scalar, vector, and tensor Poisson equations, which can be solved using standard numerical methods. Note that adding off-diagonal elements to the CFC metric in the CFC+ formalism enables us to also extract the gravitational radiation waveforms directly from metric components. These waveforms can then be compared to the ones obtained by using the Newtonian quadrupole formula.

The simulations described below demonstrate that the influence of higher order post-Newtonian terms on both the dynamics of regular supernova core collapse and also the gravitational radiation waveforms is very small. We come to the same conclusions in the case of models of rapidly rotating neutron stars. Thus, for simulating such astrophysical scenarios it is sufficient to use either the CFC or the CFC+ approximation. These yield results which are very close to each other and also to results from hydrodynamic simulations in full general relativity (see, e.g., [Shibata and Sekiguchi, 2004]).

In the following, we summarize the assumptions and approximations of our simulations of rotational core collapse and neutron star models in CFC+. More details about the specific models are given in [Cerda, et al., 2005] (see also [Dimmelmeier, et al., 2002a, Dimmelmeier, et al., 2002b] or the informations about our previous numerical simulations of general relativistic rotational core collapse.

For the rotational core collapse modes we use the same nomenclature as in our previous comprehensive parameter study of rotational core collapse in general relativity) in the CFC approximation.

The initial configurations are rotating 4/3-polytropes in equilibrium with a central density of rho_{c ini} = 10¹⁰ g/cm³ constructed by numerically solving the hydrodynamic equilibrium equations in a general relativistic spacetime [Komatsu, et al., 1989a, Komatsu, et al., 1989b]. The initial state is completely determined by the central density, the rotation rate beta_{rot ini} = E_{rot ini} / E_{pot ini}, and the rotation parameter A, which specifies the degree of differential rotation.

The equation of state during core collapse consists of a polytropic and a thermal contribution. The collapse is initiated by reducing the adiabatic exponent gamma₁. For densities rho > 2.0 ^. 10¹⁴ g/cm³, the adiabatic exponent is set to gamma₁ = 2.5 mimicking the stiffening of the equation of state above nuclear matter density.

The resuls from the simulations in CFC are given in an HTML table or a gzipped PS table.

The name of a model is determined by the value of the three free parameters according to the following table:

From that parameter space we have selected a set of four representative models, which differ in their collapse time scale and their rotational rate and profile, and accordingly show distinctive collapse behavior:

• Model A1B3G1 is a regular collapse model. Its initial model has almost uniform rotation and a moderate rotation rate beta_rot ini = 0.9%.

  With a subnuclear adiabatic index gamma₁ = 1.325 close to the initial value gamma_ini = 4 / 3, the core collapses slowly, and subsequently forms a stable remnant. This is clearly visible in the evolution of the maximum density. After the bounce, ringdown oscillations can be identified in this plot.

  The gravitational wave signal is typical for a Type I waveform, with a pronounced negative peak immediately after core bounce, and subsequent ringdown oscillations.

  The rotation plays only a minor role in the collapse dynamics. Both the density distribution, the meridional velocity vectors, and the shock front exhibit only small deviations from spherical symmetry.

  After the formation of the shock front, its outward propagation can be followed in the left box.

• Model A1B3G5 is a rapid collapse model. Its initial model has almost uniform rotation and a moderate rotation rate beta_rot ini = 0.9%.

  With a low subnuclear adiabatic index gamma₁ = 1.28, the core collapses rapidly, and subsequently forms a stable remnant. This is clearly visible in the evolution of the maximum density. After the bounce, the ringdown oscillations are strongly suppressed.

  The rotation plays only a minor role in the collapse dynamics. Both the density distribution, the meridional velocity vectors, and the shock front exhibit only small deviations from spherical symmetry.

• Model A2B4G1 is a multiple bounce collapse model. Its initial model has moderate differential rotation and a medium rotation rate beta_rot ini = 1.8%.

  With a subnuclear adiabatic index gamma₁ = 1.325 close to the initial value gamma_ini = 4 / 3, the core collapses slowly, and subsequently undergoes several phases of re-expansion and contraction. Eventually, a stable remnant will form, but on a timescale much longer than the collapse time.

  In this collapse type, rotation plays a crucial role in the collapse dynamics. Due to angular momentum conservation, the core collapse is stopped at subnuclear densities by the strong centrifugal forces.

• Model A4B5G54 is an extremely rapidly and differentially rotating collapse model. Its initial model has strong differential rotation and a high rotation rate beta_rot ini = 4.0%. As a consequence, the initial density distribution is already toroidal.

  To overcome the additional stabilization by the strong rotation, the model has a small subnuclear adiabatic index gamma₁ = 1.30. The core collapses rapidly, and spins up considerably, displaying an increasingly pronounced toroidal density distribution. It bounces at supernuclear densities, but then re-expands again to form a stable remnant at a much lower subnuclear density.

  In this collapse type, rotation has a strong influence on the collapse dynamics. While at the pole the contraction proceeds almost unaffected by centrifugal forces, it is slowed down considerably along the equator. After bounce, a strongly anisotropic shock front and large scale velocity vortices are created, leading to the formation of a short-lived accretion disk. The anisotropy of the shock front is amplified by the toroidal density distribution of the pre-shock matter.

Additional to these rotational core collapse models, we have simulated a set of uniformly rotating neutron star models at various rotation rates. They obey a simple polytropic equation of state with gamma = 2.

Analyzing the results from these numerical simulations we were able to demonstate that the second post-Newtonian CFC+ corrections do not play an important role in either the dynamics or the gravitational radiation waveforms of core collapse as well as in the case of uniformly rotating neutron stars. In all the investigated models, the effects of CFC+ in comparison to CFC were of the order of at most 10^-3. This suggests that higher order post-Newtonian corrections are completely insignificant at least on dynamic timescales. Secondly, the direct comparison of the CFC approach with exact fully general relativistic simulations of pulsating neutron stars yields normal-mode frequencies in excellent agreement. Furthermore, comparisons of the CFC approach with fully general relativistic simulations have also recently been reported by [Shibata and Sekiguchi, 2004] in the context of axisymmetric core collapse simulations. Again, the differences found in both the dynamics and the waveforms are minute, which highlights the suitability of CFC (and CFC+) for performing accurate simulations of those scenarios without the need for solving the full system of the Einstein equations.

With this investigation we have shown that a numerical code based on CFC is a very useful tool to investigate core collapse to neutron stars in general relativity. This approach is suitable to form the basis of a future core collapse supernova code which can be gradually extended in various directions to incorporate additional physics such as realistic equations of state, magnetic fields, and eventually neutrino transport. In the near future we plan to further validate the CFC+ equations in other scenarios where higher densities are present (e.g., collapse to a black hole), as well as in the fully 3D case (e.g., to investigate the merging of neutron stars). Such scenarios are in principle beyond the range of applicability of the CFC approximation, but can possibly still be handled in a satisfactory way with the new CFC+ approach.

• Cerdá-Durán, P., Faye, G., Dimmelmeier, H., Font, J.A., Ibáñez, J.M., Müller, E.,
  and Schäfer, G.,
  "CFC+: Improved dynamics and gravitational waveforms from relativistic core collapse simulations",
  Astron. Astrophys., 439, 1033-1055, (2005),
  [Article in astro-ph].


• Dimmelmeier, H., Font, J.A., and Müller, E.,
  "Gravitational waves from relativistic rotational core collapse",
  Astrophys. J. Lett., 560, L163-L166, (2001),
  [Article in astro-ph].


• Dimmelmeier, H., Font, J.A., and Müller, E.,
  "Relativistic simulations of rotational core collapse. I. Methods, initial models, and code tests",
  Astron. Astrophys., 388, 917-935, (2002),
  [Article in astro-ph].


• Dimmelmeier, H., Font, J.A., and Müller, E.,
  "Relativistic simulations of rotational core collapse. II. Collapse dynamics and gravitational radiation",
  Astron. Astrophys., 393, 523-542, (2002),
  [Article in astro-ph].


• Komatsu, H., Eriguchi, Y., and Hachisu, I.,
  "Rapidly rotating general relativistic stars - I. Numerical method and its application to uniformly rotating polytropes",
  Mon. Not. R. Astron. Soc., 237, 355-379, (1989),
  [Article in PDF format via ADS].


• Komatsu, H., Eriguchi, Y., and Hachisu, I.,
  "Rapidly rotating general relativistic stars - II. Differentially rotating polytropes",
  Mon. Not. R. Astron. Soc., 239, 153-171, (1989)
  [Article in PDF format via ADS].


• Shibata, M., and Sekiguchi, Y.,
  "Gravitational waves from axisymmetric rotating stellar core collapse to a neutron star in full general relativity",
  Phys. Rev. D, 69, 084024, (1996),
  [Article in gr-qc].


• Wilson, J.R., Mathews, G.J., and Marronetti, P.,
  "Relativistic numerical model for close neutron-star binaries",
  Phys. Rev. D, 54, 1317-1331, (1996),
  [Article in gr-qc].