A hydrodynamical perspective on shear instabilities in massive stars
Philipp Edelmann
Heidelberg Institute for Theoretical Studies, Germany
collaborators: F. Röpke (HITS), R. Hirschi (Keele), C. Georgy (Geneva), S. Jones (HITS), L. Horst (HITS)
Why are rotating stars interesting?
- in general, most stars rotate
- many show differential rotation (e.g. Kepler data)
- changes the internal structure of the star
- gives rise to many additional instabilities, mixing processes
In principle,
…we need at least 2D to describe the hydrostatic structure
…instabilities are hydrodynamic phenomena
Shellular rotation
- assumption: isobaric shells rotate on same $\Omega$
- $\rho$, $T$, … vary on shell
- Roche approximation: gravity of enclosed mass treated as of a point mass
- stellar structure equations retain 1D form
Caveats
- possible qualitatively behaviour at different latitude
- treatment of instabilities
Rotational instabilities and their treatment in 1D codes
- instabilities: dynamical shear, secular shear, GSF, ABCD,…
- usually treated as diffusion in SE codes
e.g. dynamical shear
- Richardson number $Ri=\frac{N^2}{(\partial v/\partial r)^2}$
- unstable if $Ri < Ri_c = \frac{1}{4}$
- apply $D = \frac{1}{3} r \Delta \Omega \Delta r$ in unstable zones
Challenges for multidimensional hydrodynamics
- some instabilities act on secular time scales
- differences in microphysics (e.g. EoS)
$\Rightarrow$ careful which quantities to take as input to retain same position of convection zones etc. - need to reconstruct a 3D hydrostatic model from a 1D profile
$\Rightarrow$ might lead to inconsistencies
A testbed for dynamical shear
The stellar model
- should become shear unstable in stellar evolution code
- should not show other instabilities at the same time
- should be on similar time scale in stellar evolution and hydro code
- Geneva stellar evolution code
- $20\,M_\odot$ ZAMS star, 40% crit. rotation
- post core O burning phase
- C/Ne shell interface
- convectively stable
- Ri unstable
Seven-League Hydrocode (SLH)

more information on slh-code.org
- compressible Euler equations in 1, 2, 3D
- explicit or implicit time stepping
- scales to more than 100,000 cores
- low and high Mach numbers on the same grid
(Miczek+, 2015) - arbitrary curvilinear meshes
- gravity solver
- general EoS
- nuclear reaction network
- neutrino losses
First setup
- pure hydrodynamics
- explicit time stepping
- no source terms
- Helmholtz EoS
- 2D in equatorial plane
- $512 (r) \times 1024 (\varphi)$
polar grid - 5 hours of simulated time
Evolution of Richardson number
Comparison with GENEC model
Diffusion coefficient (preliminary)
effective diffusion coefficients from averaged 2D simulations
method as in Jones+ (2016)
3D models (work in progress)
- not all latitudes necessarily stable (e.g. RT unstable)
- naïve reconstruction might lead to Solberg–Høiland instability
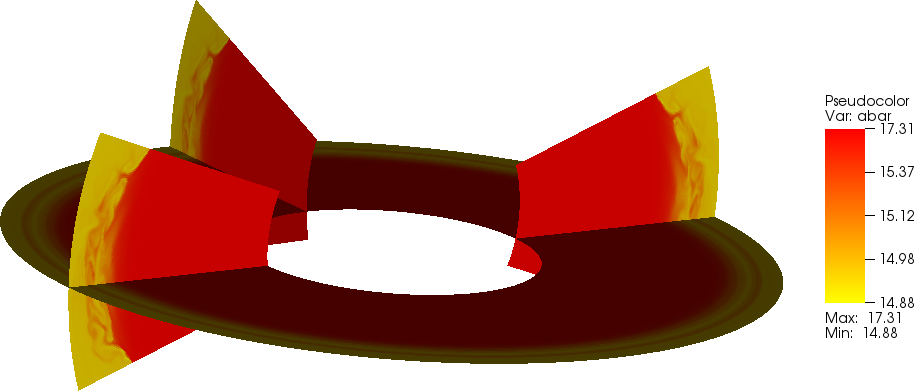
credit: L. Horst
Conclusions
- rotation is common in stars and has an important effect on stellar models
- 1D codes can treat rotation using shellular approximation
- rotational instabilities in 1D are a major uncertainty
- certain cases accessible to hydro simulations
- details of temporal evolution and extent of shear mixing differ between 1D and 2D
- effective diffusion coefficient is remarkably similar
- straightforward 3D mapping is not always consistent for all shellular rotation models
Evolution of Richardson number
Source Terms (reactions & $\nu$ losses)
Mapping from GENEC to SLH
reconstruction with $\nabla - \nabad$ from input model and additional equation for T